gaqboulder.blogg.se - Standard normal table inventory

#Standard normal table inventory license#

Average demand per day: 18,570 shirts/365 days = 51 shirts.

Maximum demand per day: 20,000 shirts/365 days = 55 shirts.

Required: Compute the reorder level and the minimum level of stock of T-shirts for Noor Clothing House.

The average lead time is 57 days and the maximum lead time is 64 days. The maximum demand is 20,000 shirts per year and the average demand is 18,570 shirts per year. Each shirt costs $15 and is sold for $20 to customers. Minimum Level of inventory = Re-order level – (Average usage × Average lead time)īoth the formulas are equivalent and produce the same result. Minimum Level of Inventory = (Maximum usage × Maximum lead time) – (Average usage × Average lead time) The formulas used to calculate the minimum level of stock are given below: If the level of stock strikes the minimum level, the management of the company must make sure that they corroborate with the supplier and take other necessary measures to make the goods (inventory or raw materials) available in time so that the business operations are not disturbed or delayed. Lead time is the expected time taken by the supplier to deliver goods at the warehouse or at the point of consumption. The minimum level of inventory is a kind of a precautionary level of inventory which indicates that the delivery of raw materials or merchandise may take more than the normal lead time.

#Standard normal table inventory license#

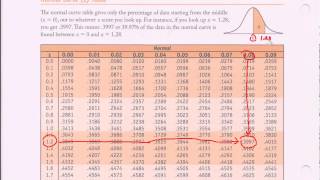
Textbook content produced by OpenStax is licensed under a Creative Commons Attribution License 4.0 license.The minimum level of stock is a certain predetermined minimum quantity of raw materials or merchandise inventory which should always be available in stock in the normal course of business. Use the information below to generate a citation. Then you must include on every digital page view the following attribution: If you are redistributing all or part of this book in a digital format, Then you must include on every physical page the following attribution: If you are redistributing all or part of this book in a print format, Want to cite, share, or modify this book? This book is The z-scores are –3 and +3 for 32 and 68, respectively. The values 50 – 18 = 32 and 50 + 18 = 68 are within three standard deviations of the mean 50. About 99.7% of the x values lie within three standard deviations of the mean.The z-scores are –2 and +2 for 38 and 62, respectively. The values 50 – 12 = 38 and 50 + 12 = 62 are within two standard deviations from the mean 50. About 95% of the x values lie within two standard deviations of the mean.The z-scores are –1 and +1 for 44 and 56, respectively.

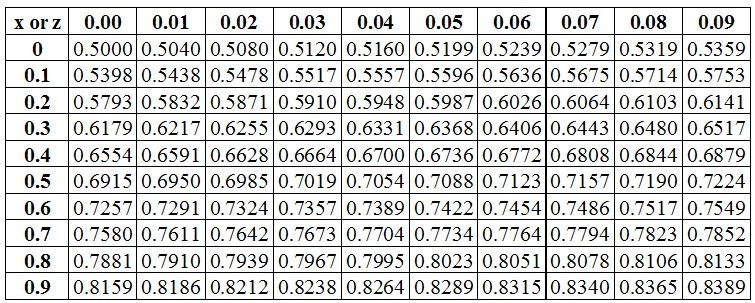
The values 50 – 6 = 44 and 50 + 6 = 56 are within one standard deviation from the mean 50. Therefore, about 68% of the x values lie between –1 σ = (–1)(6) = –6 and 1 σ = (1)(6) = 6 of the mean 50.

About 68% of the x values lie within one standard deviation of the mean.

Suppose x has a normal distribution with mean 50 and standard deviation 6. The empirical rule is also known as the 68-95-99.7 rule.

The z-scores for +3 σ and –3 σ are +3 and –3 respectively.

The z-scores for +2 σ and –2 σ are +2 and –2, respectively.

The z-scores for +1 σ and –1 σ are +1 and –1, respectively.

Notice that almost all the x values lie within three standard deviations of the mean.

About 99.7% of the x values lie between –3 σ and +3 σ of the mean µ (within three standard deviations of the mean).

About 95% of the x values lie between –2 σ and +2 σ of the mean µ (within two standard deviations of the mean).

About 68% of the x values lie between –1 σ and +1 σ of the mean µ (within one standard deviation of the mean).

The Empirical RuleIf X is a random variable and has a normal distribution with mean µ and standard deviation σ, then the Empirical Rule states the following: This score tells you that x = 10 is _ standard deviations to the _(right or left) of the mean_(What is the mean?). Suppose Jerome scores ten points in a game. Jerome averages 16 points a game with a standard deviation of four points.